- 3D-Modeling
- Digital Fabrication
- Master
- Milan
- Programming
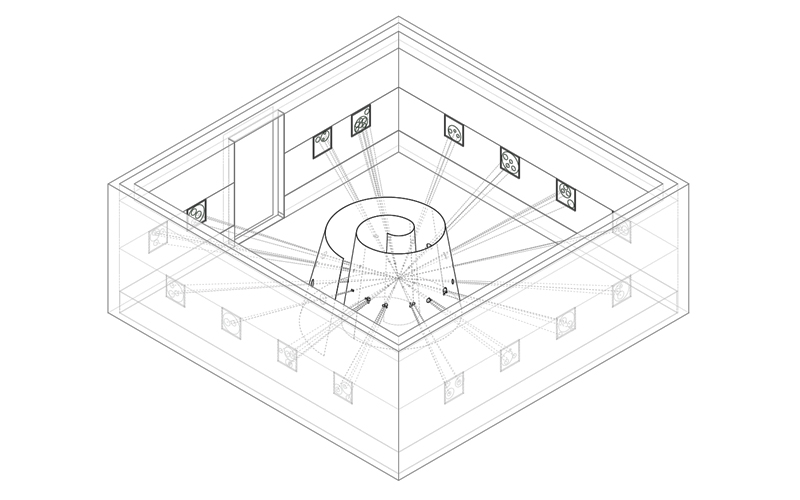
Architecture has always relied on mathematics to achieve proportioned aesthetics, structural performance, and reasonable construction. Computational tools have now given architects the means to design and build spatial concepts that would have been inconceivable even ten years ago. To inform the architectural design the project focused on the early integration of optimization parameters regarding structural performance, physical properties, and material specification as well as aspects of fabrication. The curriculum was conceived and carried out jointly by the Department of Architecture and the Department of Mathematics. The research focused on an in-depth understanding of geometric principles and their mathematical definitions as a starting point for the development of individual architectural projects. Next to the interdisciplinary exchange between architecture and mathematics, the intercultural set-up of the design teams (originating from 12 different nationalities) proved to be both challenging and inspiring throughout the process.
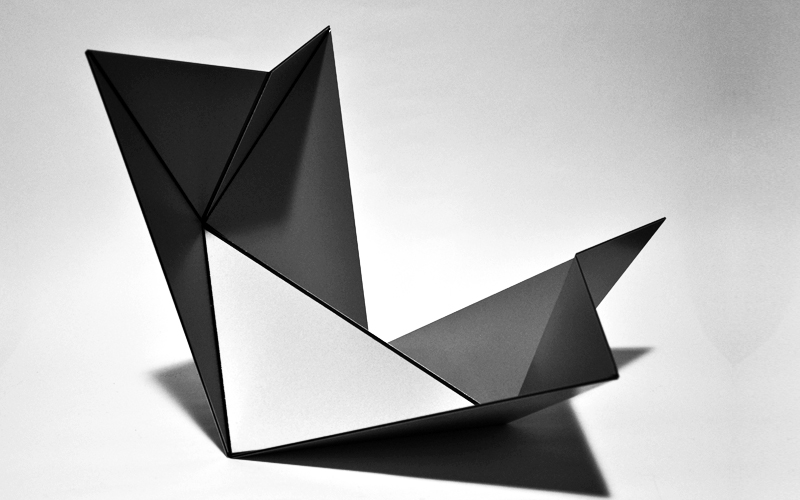
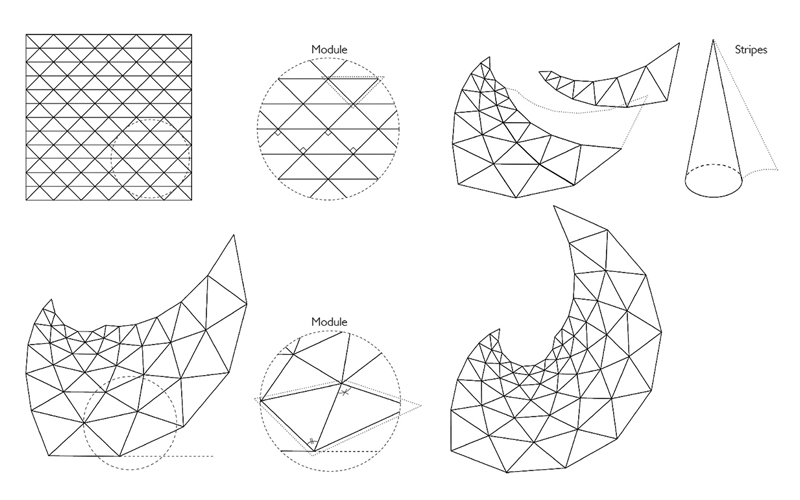
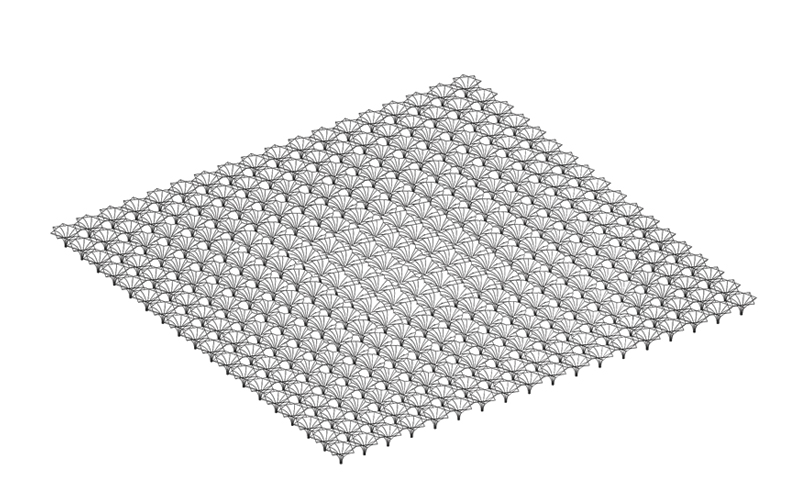
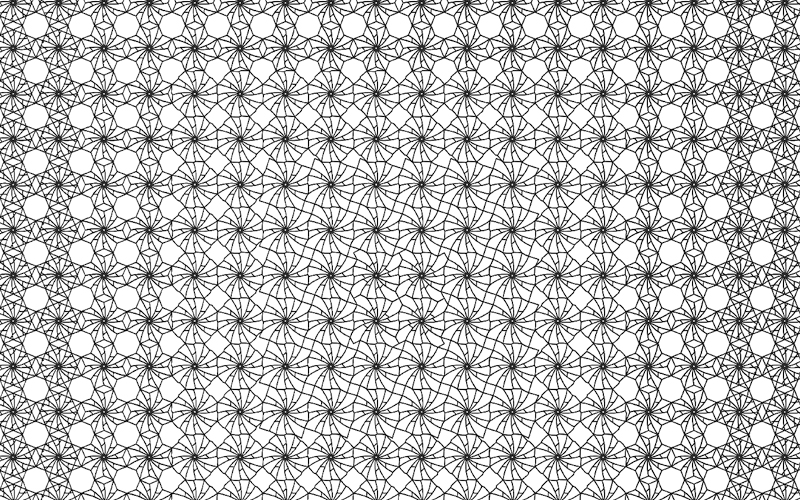
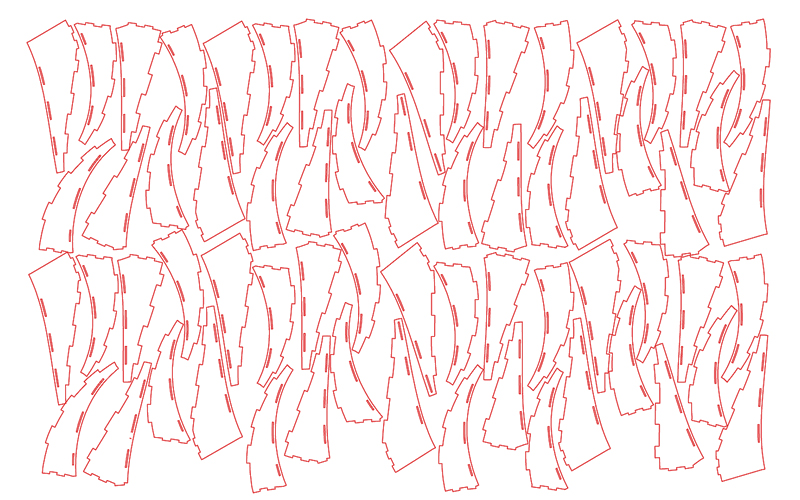
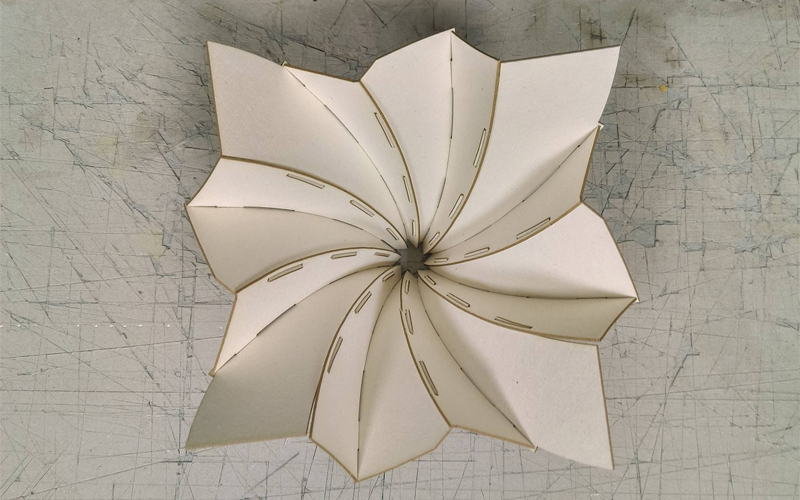
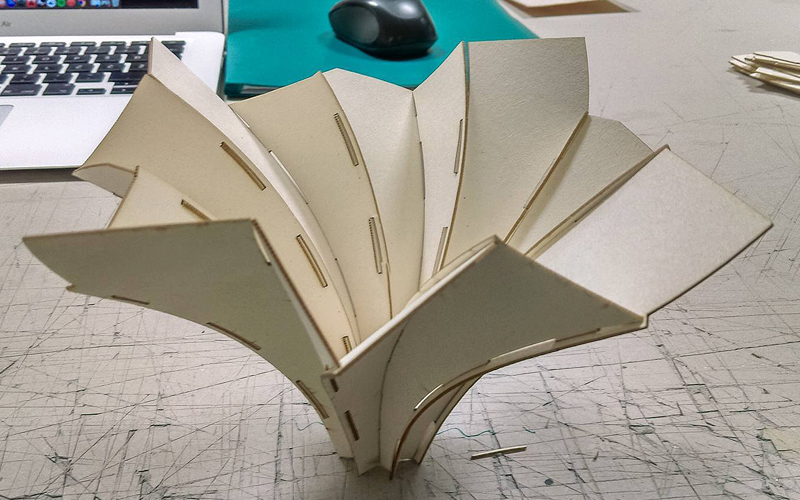
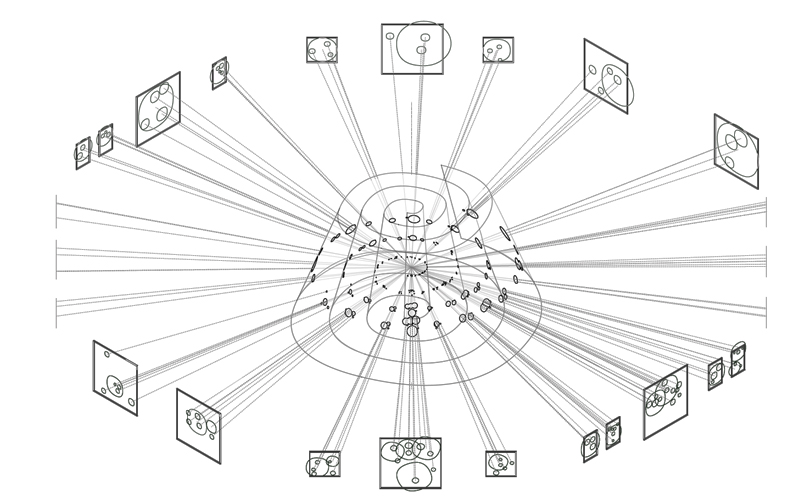
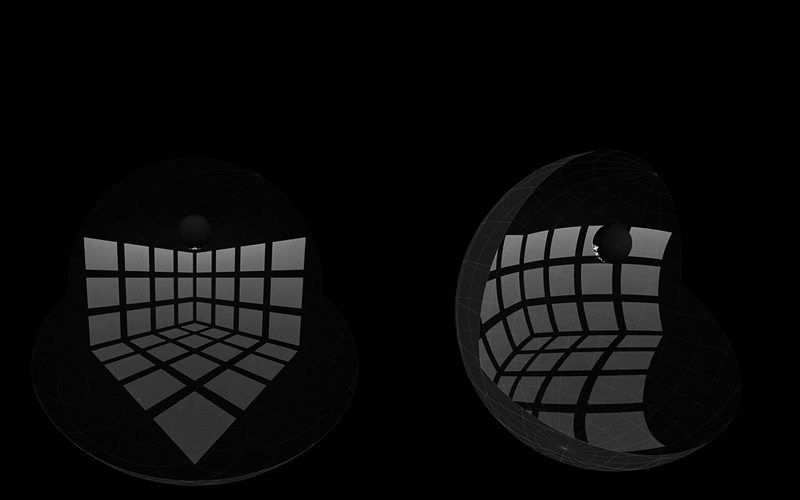
The emphasis of the course was on research-based design strategies that aimed to unfold hidden complexities of fairly simple geometric definitions. 3D-geometries are mathematical objects in the first place and as such a sequence of mathematical functions and relations used to describe a set of volumes and the surfaces that constitute their separating boundaries. CAD applications were built using these mathematical concepts but generally, do not unveil them. In order to apply these principles comprehensively , they have to be taught and understood by using the language of mathematics. Moreover, the constraints that a geometrical shape must satisfy in order to be fabricated conveniently are also described by mathematical equations. The Master course connected complexity deliberately with a bottom-up design methodology to constitute an attainable learning base for the students. As a result, almost all of the projects although starting from a fairly simple analysis of a phenomenon or mathematical problem reached a high level of complexity.
CREDITS
Steven Lane, Elena Angeli, Edoardo Corna, Milan Dragoljevic, Federico lannarone, Martina Jamroz, Alfiia Khabibullina, Federica Giorgetta, Alberto Lunardi, Alessio Palmieri, Matija Solomun, Felix Obermair.
Marco Hemmerling, Carlo de Falco, Alessio Mazzucchi, Jacopo Corno
Politecnico di Milano